
Free Download Remedial Mathematics Notes in pdf – Bpharm 1st Semester. High quality, well-structured and Standard Notes that are easy to remember.
Welcome to Pharmdbm.com
Pharmdbm provides standard or well-structured Notes for Bpharm students. The notes are free to download. Each semester notes of Bpharm are available on www.pharmdbm.com.
In this post you can download notes of Remedial Mathematics (BP106RMT). All units are available to download for free.
Remedial Mathematics Notes Unit 1 – 5
UNIT – 1
Partial fraction, Logarithms, Function, Limits and continuity

UNIT – 2
Matrices and Determinant
UNIT – 3
Calculus – Differentiation
UNIT – 4
Analytical Geometry – Straight line, Integration
UNIT – 5
Differential Equations, Laplace Transform
Syllabus of Remedial Mathematics – Bpharm 1st Semester
UNIT – 1
Partial fraction:
Introduction, Polynomial, Rational fractions, Proper and Improper fractions,
Partial fraction , Resolving into Partial fraction, Application of Partial
Fraction in Chemical Kinetics and Pharmacokinetics
Logarithms:
Introduction, Definition, Theorems/Properties of logarithms, Common
logarithms, Characteristic and Mantissa, worked examples, application of
logarithm to solve pharmaceutical problems.
Function:
Real Valued function, Classification of real valued functions,
Limits and continuity :
Introduction , Limit of a function, Definition of limit of a function
UNIT – 2
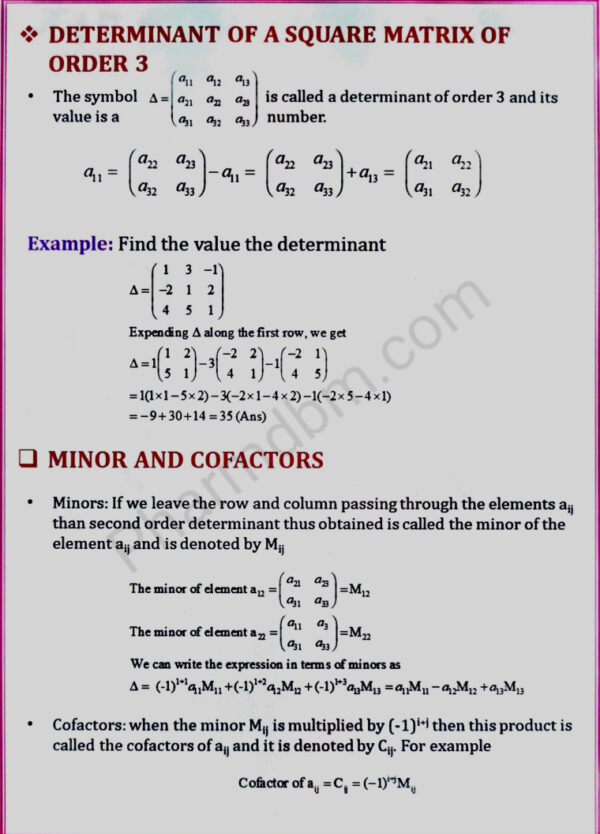
Matrices and Determinant:
Introduction matrices, Types of matrices, Operation on matrices,
Transpose of a matrix, Matrix Multiplication, Determinants, Properties of
determinants , Product of determinants, Minors and co-Factors, Adjoint
or adjugate of a square matrix , Singular and non-singular matrices,
Inverse of a matrix, Solution of system of linear of equations using matrix
method, Cramer’s rule, Characteristic equation and roots of a square
matrix, Cayley–Hamilton theorem,Applicationof Matrices in solving
Pharmacokinetic equations
UNIT – 3
Calculus
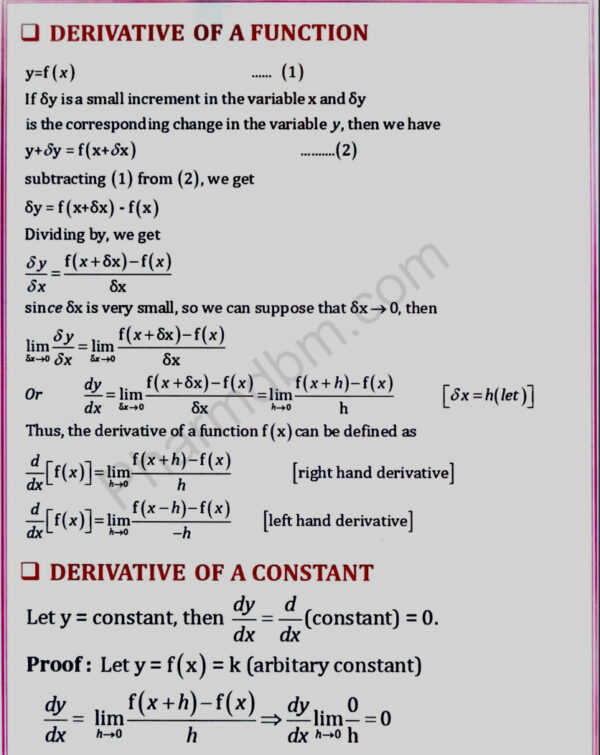
Differentiation : Introductions, Derivative of a function, Derivative of a
constant, Derivative of a product of a constant and a function , Derivative
of the sum or difference of two functions, Derivative of the product of two
functions (product formula), Derivative of the quotient of two functions
(Quotient formula) – Without Proof,
UNIT – 4
Analytical Geometry
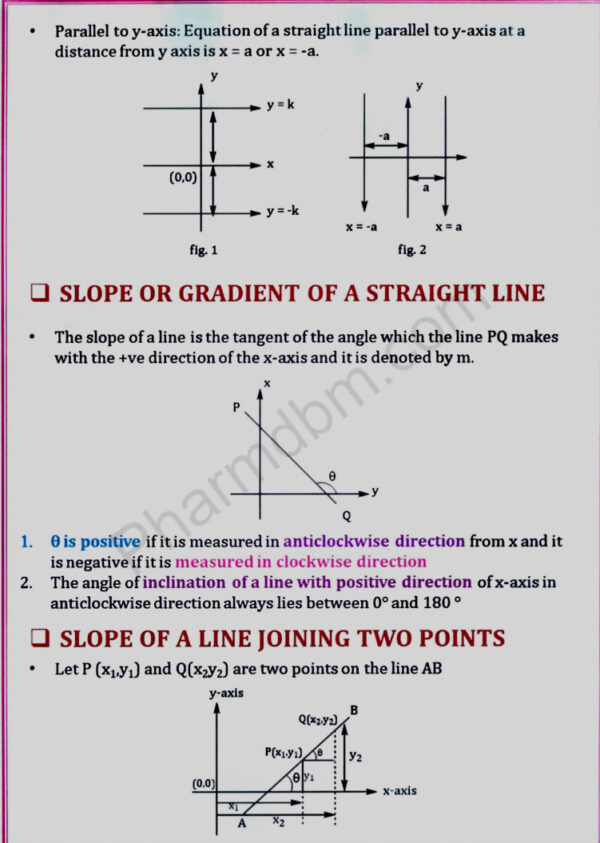
Introduction: Signs of the Coordinates, Distance formula,
Straight Line : Slope or gradient of a straight line, Conditions for
parallelism and perpendicularity of two lines, Slope of a line joining two
points, Slope – intercept form of a straight line
Integration:
Introduction, Definition, Standard formulae, Rules of integration , Method of
substitution, Method of Partial fractions, Integration by parts, definite
integrals, application
UNIT – 5
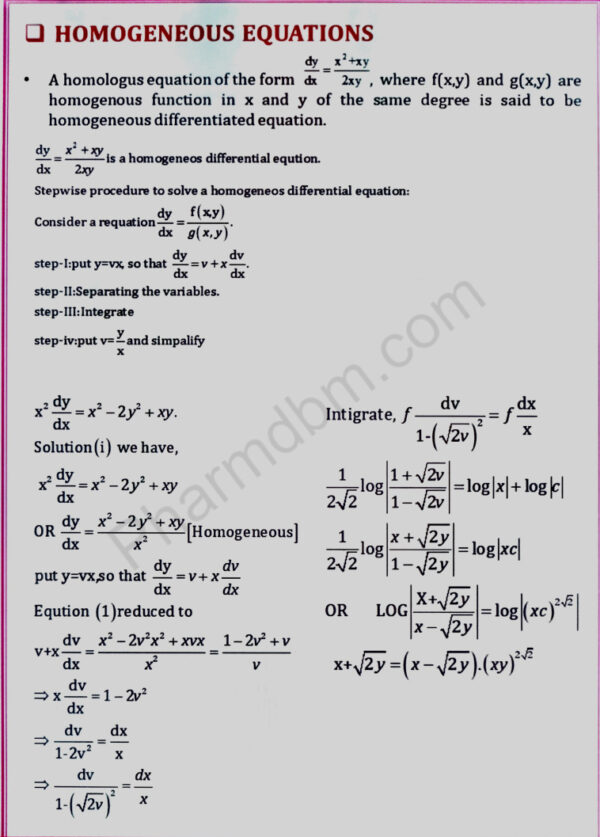
Differential Equations : Some basic definitions, Order and degree, Equations in separable form , Homogeneous equations, Linear
Differential equations, Exact equations, Application in solving
Pharmacokinetic equations
Laplace Transform : Introduction, Definition, Properties of Laplace
transform, Laplace Transforms of elementary functions, Inverse
Laplace transforms, Laplace transform of derivatives, Application to
solve Linear differential equations, Application in solving Chemical
kinetics and Pharmacokinetics equations
Scope of Remedial Mathematics
This is an introductory course in mathematics. This subject deals with the
introduction to Partial fraction, Logarithm, matrices and Determinant, Analytical
geometry, Calculus, differential equation and Laplace transform
Objectives of Remedial Mathematics
Upon completion of the course the student shall be able to:-
- Know the theory and their application in Pharmacy.
- Solve the different types of problems by applying theory.
- Appreciate the important application of mathematics in Pharmacy.
